The cosine function ‘or’ Cos Theta is one of the three most common trigonometric functions along with sine and tangent. In right-angled trigonometry, the cosine function is defined as the ratio of the adjacent side and hypotenuse.
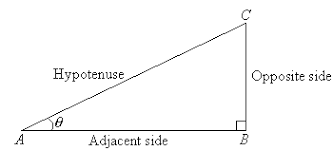
The mathematical denotation of the sine function is,
\(\cos(\theta) = \frac{\text{Adjacent Side}}{\text{Hypotenuse Side}}\)Index
More About Cos Theta
Cos theta formula can also be calculated from the product of the tangent of the angle with the sine of the angle.
\(\cos(\theta) = \frac{\sin(\theta)}{ \tan(\theta)}\)The derivative of \(\cos(\theta)\) in calculus is \(-\sin(\theta)\) and the integral of it is \(\sin(\theta)\). The reciprocal of cos theta is sec theta.
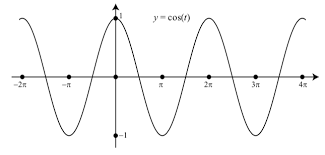
Below is a table of cos theta values for different degrees and radians.
| Radians | Degree | Tangent Value |
| 0 | 0° | 0 |
| \(\frac{\pi}{6}\) | 30° | \(\frac{\sqrt{3}}{2}\) |
| \(\frac{\pi}{4}\) | 45° | \(\frac{1}{\sqrt{2}}\) |
| \(\frac{\pi}{3}\) | 60° | \(\frac{1}{2}\) |
| \(\frac{\pi}{2}\) | 90° | 0 |
| \(\pi\) | 180° | -1 |
| \(\frac{3\pi}{2}\) | 270° | 0 |
| \(2\pi\) | 360° | 1 |
Important Cos Theta Formula
Some important properties of the cosine function and cos theta formula are:
- \(\cos(-x) = -\cos(x)\)
- \(\cos(90° – x) = \sin(x)\)
- \(\cos(x + 2\pi) = \cos(x)\)
- \(\cos(\pi – x) = -\cos(x)\)
- \(\cos^2(x) + \sin^2(x) = 1\)
- \(cos(x + y) = \cos(x)*\cos(y) + \sin(x)*\sin(y)\)
- \(\cos(x – y) = \cos(x)*\cos(y) + \sin(x)\sin(y)\)
- \(\cos(2x) = \cos^2(x) – \sin^2(x)\)
- \(\cos(3x) = 4\cos^3(x) – 3\cos(x)\)
- \(\cos(\frac{x}{2}) = \pm \sqrt{\frac{1 + \cos(x)}{2}}\)
- \(\cos(x) + \cos(y) = 2 \cos(\frac{x + y}{2}) \cos(\frac{x – y}{2})\)
- \(\cos(x) – \cos(y) = -2 \sin(\frac{x + y}{2}) \sin(\frac{x – y}{2})\)
- \(\cos(x) = \frac{1 – \tan^2(\frac{x}{2})}{1 + \tan^2(\frac{x}{2})}\)
Solved Examples
Question 1. If \(\sin(x) = \frac{6}{13}\), calculate the value of \(\cos(x)\).
Solution. Using trigonometric identity,
\(\cos^2(x) = 1 – \sin^2(x) = 1 – \frac{36}{169}\)
\(\cos^2(x) = \frac{133}{169}\)
\(∴ \cos(x) = \frac{\sqrt{133}}{13}\)
Question 2. If \(\tan(\frac{x}{2}) = \frac{5}{8}\), calculate the value of \(\cos(x)\).
Solution. Using trigonometric identity,
\(\begin{align}
\cos(x) & = \frac{1 – \tan^2(\frac{x}{2})}{1 + \tan^2(\frac{x}{2})}\\
& = \frac{1 – (\frac{5}{8})^2}{1 + (\frac{5}{8})^2}\\
& = \frac{39/64}{89/64}\\
\cos(x) & = \frac{80}{89}\\
\end{align}
\)
Question 3. Consider a ladder leaning against a brick wall making an angle of 60o with the horizontal. If the ground distance between the ladder and the wall is 10 ft, then up to what height of the wall the ladder reaches?
Solution. Given, Angle between ladder and ground, \(\theta = 60\) and distance from ladder to wall = 10 ft.
As we know,
\(\cos(\theta) = \frac{\text{Adjacent}}{\text{Hypotenuse}}\)
\(\cos(60°) = \frac{\text{10}}{Hypotenuse}\)
\(\frac{1}{2} = \frac{\text{10}}{Hypotenuse}\)
\(\text{Hypotenuse} = 20\)
∴ The height of the wall up to which the ladder reaches is 20 ft.
FAQs
As we know, the angle (-x) lies in the 4th quadrant of a graph, and cosine is positive in this quadrant. Hence, this shows that cos(-x) = cos(x).
Sin theta of a right-angled triangle is equal to the ratio of the length of the adjacent side to the length of the hypotenuse.
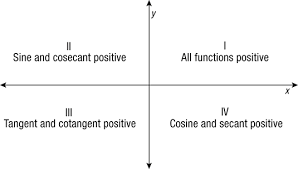
It can be observed from the above graph that cosine function is positive in the 1st and 4th quadrants and negative in the 2nd and 3rd quadrants.
More Trigonometric Functions
