Pi, denoted by π, is a constant that we’ve been using since our fifth grade when we first learnt how to calculate an area enclosed by a circle. Do you know the exact value of Pi? Actually, none of us knows it as it is endless. Yes, the decimals after 3 in pi extend till infinity.
To make calculations simple, the fractional value of Pi is most widely used, which is 22/7. The decimal value of Pi is 3.14.
Index
History
Pi (π) has been known for almost 4000 years—but even if we calculated the number of seconds in those 4000 years and calculated π to that number of places, we would still only be approximating its actual value.
Mathematicians began using the Greek letter π in the 1700s. Introduced by William Jones in 1706, use of the symbol was popularized by Leonhard Euler, who adopted it in 1737.
The earliest known use of the Greek letter π to represent the ratio of a circle’s circumference to its diameter was by Welsh mathematician William Jones in 1706.

Definition of Pi
Pi is a constant. It is often referred to as the “Archimedes’s constant”. It means that its value will be the same no matter what the conditions are. Pi, one of the most well-known mathematical constants, is the ratio of a circle’s circumference to its diameter.
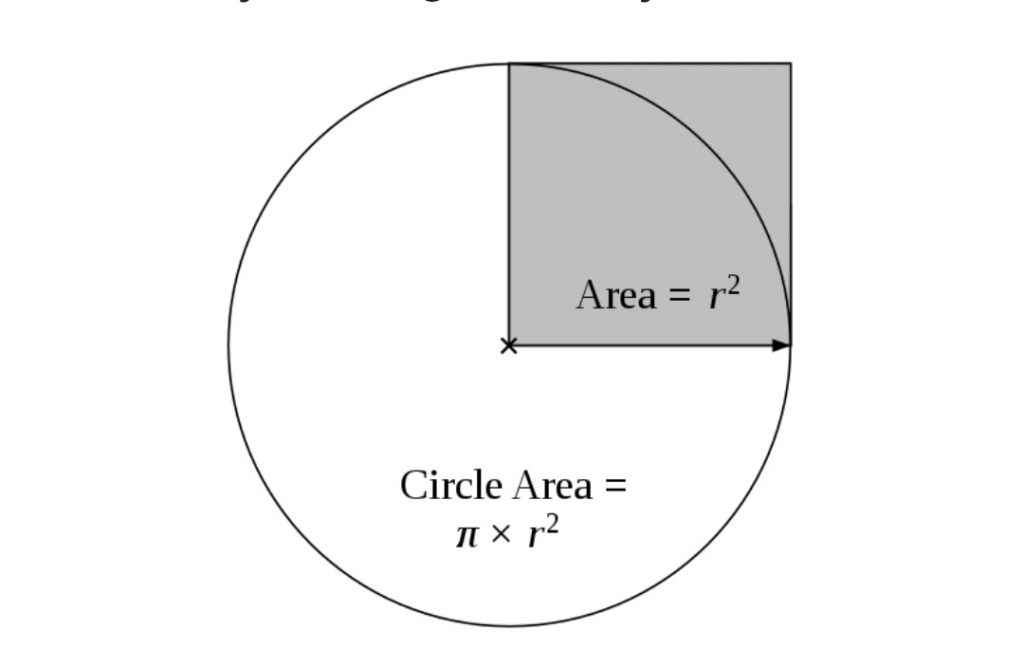
Formulae Involving Pi
Because π is closely related to the circle, it is found in many formulae from the fields of geometry and trigonometry, particularly those concerning circles, spheres, or ellipses. Other branches of science, such as statistics, physics, Fourier Analysis, and Number Theory, also include π in some of their important formulae.
π appears in formulae for areas and volumes of geometrical shapes based on circles, such as ellipses, spheres, cones, and tori. Below are some of the more common formulae that involve π.
- The circumference of a circle with radius r is 2πr.
- The area of a circle with radius r is πr2.
- The volume of a sphere with radius r is 4/3πr3.
- The surface area of a sphere with radius r is 4πr2.
Applications of Pi
Pi is used in various fields of mathematics including trigonometry, euclidean geometry etc.
It is used in the fields of Physics like mechanics, electricity, magnetism, gravitation, modern physics.
Since π is can be converted to degrees, we can find cosine, sine and tan of π
- sin of pi – sin(π) can be written as sin(180°) and the value comes out to be 0.
- cos of pi – cos(π) is equal to Negative 1.
- tan of pi – tan(π) gives the value positive 1.
Solved Problems
Question 1. Evaluate an area enclosed by a circle of radius 3m. (Take value of pi (π) = 3.14)
Answer. According to the formula,
Area enclosed by a circle = πR2 where R is the radius of the circle
=> A = π(3m)2
Taking the value of pi (π) as 3.14,
A = 3m2 × 3.14 = 9.42 m2
Question 2. Find the volume of a sphere of radius 4m (Take value of pi (π) as 3)
Answer. Using the formula,
V = 4/3πR3
Taking the value of pi (π) as 3 and radius as 4.
V = 4/3 × 3 × 4×4×4 m3
Volume of the sphere comes out to be 256m3
FAQs
The value of Pi can be taken as 3.14 for simpler calculations. It’s fractional value is 22/7. We often take pi as 180° only if π is expressed in radians.
The constant value of pi was discovered in 250 B.C.
Around 250 B.C., the Greek mathematician Archimedes calculated the ratio of a circle’s circumference to its diameter.
Pi is an irrational number – you can’t write it down as a non-infinite decimal. This means you need an approximate value of Pi. The decimal points extend till infinity
3.1415926535 8979323846 2643383279 5028841971 6939937510 5820974944 5923078164 0628620899 8628034825 3421170679 …
